
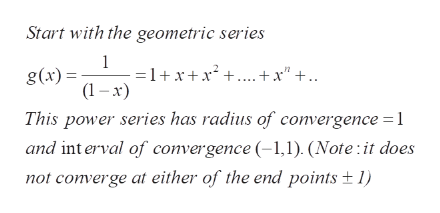
You will discover exactly the recurrence you found. The power series n 0 a n x n is an infinite series, and looks like a function of x. It also gives a plot of approximation of x up to certain. Your equation will work the same way: Multiply through by the denominator, gather like terms, and then equate the coefficients on the left with those on the right. Find the power series expansion and representation using the power series calculator with steps. We can just shift the center of our power series if we want to approximate a value outside the interval of convergence. Usually, some power series arise from derivatives. Find a power series representation for g(x) 1 x + 3 and state the interval of convergence. While it would be easy to say that 1 (1 x)2 ( k0xk)2 This is not a valid representation of a power series. Find the power series representation for the follwoing function centered at a 0 and specify its radisu of convergence. functions derivatives evaluated at the series center.

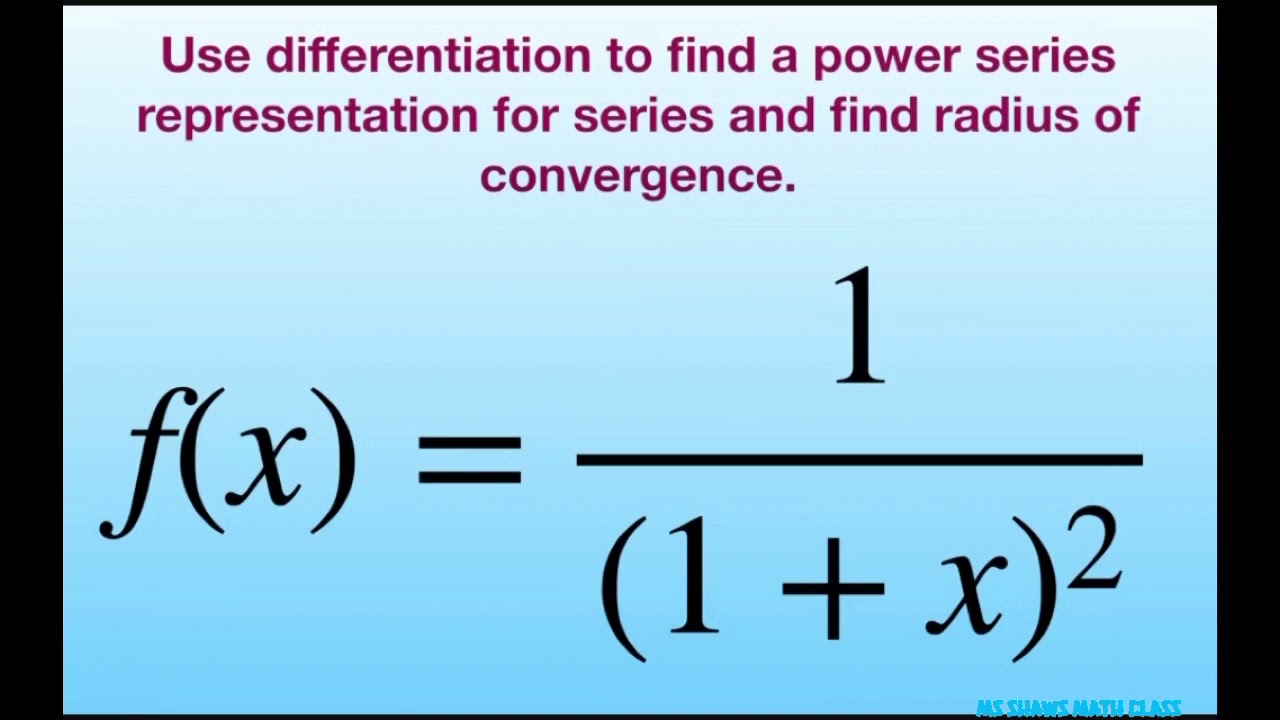
#DFIND A POWER SERIES REPERESENTATION HOW TO#
k0xk The radius of convergence for this power series is x ( 1,1). Find out how to watch the Power shows in order, as well as where to watch. (Please see edit below I originally asked how to find a power series expansion of a given function, but I now wanted to know how to do the reverse case.)Ĭan someone please explain how to find the power series expansion of the function in the title? Also, how would you do it in the reverse case? That is, given the power series expansion, how can you deduce the function $\frac=1+1x+2x^2+3x^3+5x^4+8x^5+13x^6+\ldots$$ This is fairly similar to 1 1 x, for which we know a power series: 1 1 x 1 + x + x2 +.


 0 kommentar(er)
0 kommentar(er)
